FINITE IMPULSE RESPONSE & INFINITE IMPULSE RESPONSE FILTERS EXPLAINED.
Today we look at FIR (Finite Impulse Response) and IIR (Infinite Impulse Response) filters, what they are, and how this understanding can impact your mixes!
Firstly, I think a brief overview of equalisation is needed to contextualise the tasks that IIR and FIR filters perform…
A little bit about equalisation…
Analog and digital EQs use a series of minuscule delays at specific frequencies - know as phase shift to achieve the audible outcomes that we associate with equalisation.
As we have previously mentioned, phase (or polarity) refers to the relationship of wave cycles between two or more audio signals. It can have a variety of sonic implications for processing chains. In this instance, it largely refers to a boost or cut to particular frequencies within a signal.
In the analog realm, phase shift (and frequency response) are shaped by the use of capacitance, resistance & inductance. The delayed signal is combined with the original - causing phase cancellation and a non-flat frequency response.
In the digital realm, phase shift is created by the use of digital delays. A “digital delay line” is a discrete series of equally-spaced number addresses (or taps) that are mathematically related to sample rate.
As an audio signal is fed into the processor, each impulse is sequentially passed through the digital delay line, affecting phase and thus the spectral aspects of the signal. The affected audio is summed with the original signal and they pass through the EQ’s output.
So what’s an Impulse Response?
In digital signal processing, an impulse refers to a brief input signal fed into a processing unit. In the context of equalisation, this refers to isolated transients of acoustic or electric audio signals.
Therefore, in the context of FIR/IIR audio processing, the term “impulse response” refers to an equaliser’s ability to spectrally react accordingly to individual harmonic impulses fed to its input.
The terms “finite” and “infinite” relate to feedback structure, and are key to understanding this next stage…
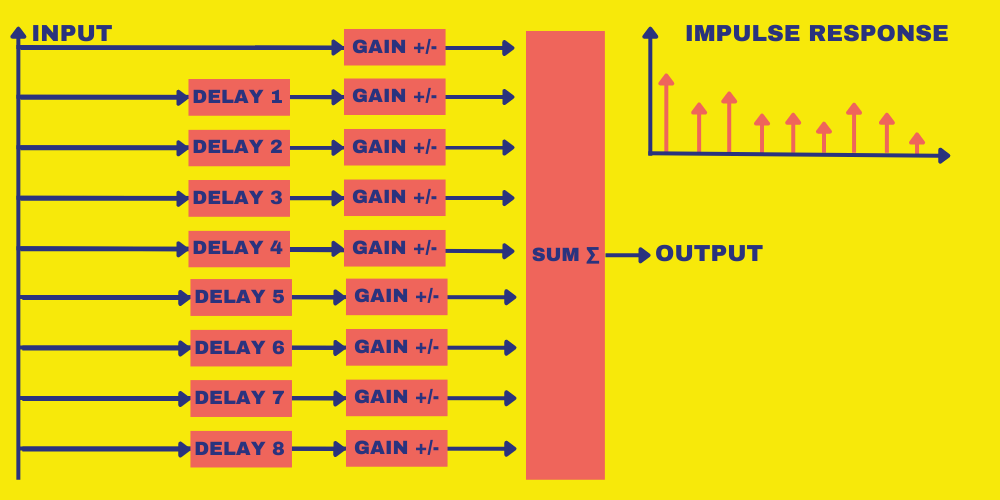
Finite Impulse Response (FIR)
“FIR filters are also known as non-recursive filters, convolution filters, or moving-average filters because the output values of an FIR filter are described as a finite convolution.”
A convolution is form of audio signal processing, consisting of three operations:
Using phase shift to delay an audio signal by a fixed number of samples.
Applying amplification (boosting) or attenuation (cutting) to the delayed audio.
Summing the delayed and amplified/attenuated signal with the input signal.
If the term “convolution” sounds familiar, you may well have encountered its use in programmes such as Ableton’s Convolution Reverb. We will delve into this application of impulse responses in the emulation of real acoustic reverberance in a later article!
FIR processing filters its audio input by feeding the signal into a delay line. The signal runs sequentially through this delay line, and its signal amplitude is proportionately boosted or cut and summed with the unaffected original signal.
The output signal from an FIR filter is shaped only by the present and past input data. The output does not depend on past output values, so the impulse response decays to zero and resets over a finite time-frame.
Because of this, FIR filters can:
Achieve linear phase response.
Prevent phase smearing as the phase shift is spectrally consistent.
Provide more stable processing as they do not depend on past output information feedback like IIR processing.
Because of this, FIR filters are often used when signal filtering must be free from the consequences of phase. They can be found in linear phase EQs, anti-aliasing filters and reconstruction filters in A/D - D/A converters, as well as sample rate converters.
Infinite Impulse Response (IIR)
The defining difference between Infinite and Finite Impulse Responses is that with IIR, the delay line processes output values and feeds them back into the input of the delay chain.
IIR filters are defined by an internal feedback mechanism, which operates for an indefinite (or theoretically infinite) period of time.
“The output values of IIR filters are calculated by adding the weighted sum of previous and current input values to the weighted sum of previous output values.”
Because of this, IIR filters:
Operate using significantly less processing power as they require fewer coefficients than FIR processing.
Generally lend to minimal-phase equalisation as they have non-linear phase characteristics.
Can be emulated in the analog realm (there is no analog equivalent for FIR filtering).
Concluding remarks
The sonic impact of phase shift is up for debate. Some engineers swear by IIR / linear phase processing, whilst some claim that phase shift and smearing from equalisation is unnoticeable. Some suggest that the artefacts others claim to be a result of phase shift, are in fact caused by comb filtering during the recording stage, and equalisation is simply making these issues more noticeable.
So what does this mean for your DAW projects?
My advice would be to experiment with your available options. If you are producing inside the box, there is a good chance that you will not be working with any audio recorded using stereo mic techniques. Therefore, you may never encounter some of the polarity issues that linear phase equalisation is designed to negate.
That said, there are certainly instances where I would still experiment with FIR processing, such as:
Mastering.
Parallel or buss processing.
It is a misconception that linear phase processing intrinsically improves an audio signal’s sonic qualities. This is not the case. Treat each audio instance as unique, trust your ears, and see FIR/IIR processing as equally valuable tools in your audio engineering belt when used appropriately.
Article Sources / Further Reading:Advanced Solutions Nederlands. (2020). Difference between IIR and FIR filters: a practical design guide. [Online]. www.advsolned.com. Last Updated: 28/04/2020. Available at: https://www.advsolned.com/difference-between-iir-and-fir-filters-a-practical-design-guide/ [Accessed 5 February 2023].Juan S Audio. (2021). IIR vs FIR: Understanding their differences. [Online]. www.juansaudio.com. Last Updated: 08/07/2021. Available at: https://www.juansaudio.com/post/iir-vs-fir-understanding-their-differences [Accessed 5 February 2023].McFee, Brian. (2020). Digital Signals Theory. [Online]. https://brianmcfee.net. Last Updated: Publish Date Unknown. Available at: https://brianmcfee.net/dstbook-site/content/ch03-convolution/Building.html [Accessed 5 February 2023].Michael John. (Publish Date Unknown). The Complete FIR Filter Guide. [Online]. https://eclipseaudio.com. Last Updated: Publish Date Unknown. Available at: https://eclipseaudio.com/fir-filter-guide/ [Accessed 5 February 2023].NI. (2022). IIR Filters and FIR Filters. [Online]. https://www.ni.com. Last Updated: 01/08/2022. Available at: https://www.ni.com/docs/en-US/bundle/diadem/page/genmaths/genmaths/calc_filterfir_iir.htm [Accessed 5 February 2023]. Winer, Ethan. (Publish Date Unknown). EQ Phase. [Online]. https://ethanwiner.com. Last Updated: Publish Date Unknown. Available at: https://ethanwiner.com/EQPhase.html [Accessed 5 February 2023].